Notation for the (principal) square root of x.
For example, √25 = 5, since 25 = 5 ⋅ 5, or 52 (5 squared).
In mathematics, a square root of a number x is a number y such that y2 = x; in other words, a number y whose square (the result of multiplying the number by itself, or y ⋅ y) is x.[1] For example, 4 and −4 are square roots of 16, because 42 = (−4)2 = 16.
Every nonnegative real number x has a unique nonnegative square root, called the principal square root, which is denoted by 


Every positive number x has two square roots: 


Square roots of negative numbers can be discussed within the framework of complex numbers. More generally, square roots can be considered in any context in which a notion of the «square» of a mathematical object is defined. These include function spaces and square matrices, among other mathematical structures.
History
The Yale Babylonian Collection YBC 7289 clay tablet was created between 1800 BC and 1600 BC, showing 

The Rhind Mathematical Papyrus is a copy from 1650 BC of an earlier Berlin Papyrus and other texts – possibly the Kahun Papyrus – that shows how the Egyptians extracted square roots by an inverse proportion method.[6]
In Ancient India, the knowledge of theoretical and applied aspects of square and square root was at least as old as the Sulba Sutras, dated around 800–500 BC (possibly much earlier).[citation needed] A method for finding very good approximations to the square roots of 2 and 3 are given in the Baudhayana Sulba Sutra.[7] Aryabhata, in the Aryabhatiya (section 2.4), has given a method for finding the square root of numbers having many digits.
It was known to the ancient Greeks that square roots of positive integers that are not perfect squares are always irrational numbers: numbers not expressible as a ratio of two integers (that is, they cannot be written exactly as 
The particular case of the square root of 2 is assumed to date back earlier to the Pythagoreans, and is traditionally attributed to Hippasus.[citation needed] It is exactly the length of the diagonal of a square with side length 1.
In the Chinese mathematical work Writings on Reckoning, written between 202 BC and 186 BC during the early Han Dynasty, the square root is approximated by using an «excess and deficiency» method, which says to «…combine the excess and deficiency as the divisor; (taking) the deficiency numerator multiplied by the excess denominator and the excess numerator times the deficiency denominator, combine them as the dividend.»[9]
A symbol for square roots, written as an elaborate R, was invented by Regiomontanus (1436–1476). An R was also used for radix to indicate square roots in Gerolamo Cardano’s Ars Magna.[10]
According to historian of mathematics D.E. Smith, Aryabhata’s method for finding the square root was first introduced in Europe by Cataneo—in 1546.
According to Jeffrey A. Oaks, Arabs used the letter jīm/ĝīm (ج), the first letter of the word «جذر» (variously transliterated as jaḏr, jiḏr, ǧaḏr or ǧiḏr, «root»), placed in its initial form (ﺟ) over a number to indicate its square root. The letter jīm resembles the present square root shape. Its usage goes as far as the end of the twelfth century in the works of the Moroccan mathematician Ibn al-Yasamin.[11]
The symbol «√» for the square root was first used in print in 1525, in Christoph Rudolff’s Coss.[12]
Properties and uses
The graph of the function f(x) = √x, made up of half a parabola with a vertical directrix
The principal square root function 
The square root of x is rational if and only if x is a rational number that can be represented as a ratio of two perfect squares. (See square root of 2 for proofs that this is an irrational number, and quadratic irrational for a proof for all non-square natural numbers.) The square root function maps rational numbers into algebraic numbers, the latter being a superset of the rational numbers).
For all real numbers x,
(see absolute value)
For all nonnegative real numbers x and y,
and
The square root function is continuous for all nonnegative x, and differentiable for all positive x. If f denotes the square root function, whose derivative is given by:
The Taylor series of 
The square root of a nonnegative number is used in the definition of Euclidean norm (and distance), as well as in generalizations such as Hilbert spaces. It defines an important concept of standard deviation used in probability theory and statistics. It has a major use in the formula for roots of a quadratic equation; quadratic fields and rings of quadratic integers, which are based on square roots, are important in algebra and have uses in geometry. Square roots frequently appear in mathematical formulas elsewhere, as well as in many physical laws.
Square roots of positive integers
A positive number has two square roots, one positive, and one negative, which are opposite to each other. When talking of the square root of a positive integer, it is usually the positive square root that is meant.
The square roots of an integer are algebraic integers—more specifically quadratic integers.
The square root of a positive integer is the product of the roots of its prime factors, because the square root of a product is the product of the square roots of the factors. Since 
As decimal expansions
The square roots of the perfect squares (e.g., 0, 1, 4, 9, 16) are integers. In all other cases, the square roots of positive integers are irrational numbers, and hence have non-repeating decimals in their decimal representations. Decimal approximations of the square roots of the first few natural numbers are given in the following table.
-
n truncated to 50 decimal places
0 0 1 1 2 1.41421356237309504880168872420969807856967187537694 3 1.73205080756887729352744634150587236694280525381038 4 2 5 2.23606797749978969640917366873127623544061835961152 6 2.44948974278317809819728407470589139196594748065667 7 2.64575131106459059050161575363926042571025918308245 8 2.82842712474619009760337744841939615713934375075389 9 3 10 3.16227766016837933199889354443271853371955513932521
As expansions in other numeral systems
As with before, the square roots of the perfect squares (e.g., 0, 1, 4, 9, 16) are integers. In all other cases, the square roots of positive integers are irrational numbers, and therefore have non-repeating digits in any standard positional notation system.
The square roots of small integers are used in both the SHA-1 and SHA-2 hash function designs to provide nothing up my sleeve numbers.
As periodic continued fractions
One of the most intriguing results from the study of irrational numbers as continued fractions was obtained by Joseph Louis Lagrange c. 1780. Lagrange found that the representation of the square root of any non-square positive integer as a continued fraction is periodic. That is, a certain pattern of partial denominators repeats indefinitely in the continued fraction. In a sense these square roots are the very simplest irrational numbers, because they can be represented with a simple repeating pattern of integers.
-
= [1; 2, 2, …] = [1; 1, 2, 1, 2, …] = [2] = [2; 4, 4, …] = [2; 2, 4, 2, 4, …] = [2; 1, 1, 1, 4, 1, 1, 1, 4, …] = [2; 1, 4, 1, 4, …] = [3] = [3; 6, 6, …] = [3; 3, 6, 3, 6, …] = [3; 2, 6, 2, 6, …] = [3; 1, 1, 1, 1, 6, 1, 1, 1, 1, 6, …] = [3; 1, 2, 1, 6, 1, 2, 1, 6, …] = [3; 1, 6, 1, 6, …] = [4] = [4; 8, 8, …] = [4; 4, 8, 4, 8, …] = [4; 2, 1, 3, 1, 2, 8, 2, 1, 3, 1, 2, 8, …] = [4; 2, 8, 2, 8, …]
The square bracket notation used above is a short form for a continued fraction. Written in the more suggestive algebraic form, the simple continued fraction for the square root of 11, [3; 3, 6, 3, 6, …], looks like this:
where the two-digit pattern {3, 6} repeats over and over again in the partial denominators. Since 11 = 32 + 2, the above is also identical to the following generalized continued fractions:
Computation
Square roots of positive numbers are not in general rational numbers, and so cannot be written as a terminating or recurring decimal expression. Therefore in general any attempt to compute a square root expressed in decimal form can only yield an approximation, though a sequence of increasingly accurate approximations can be obtained.
Most pocket calculators have a square root key. Computer spreadsheets and other software are also frequently used to calculate square roots. Pocket calculators typically implement efficient routines, such as the Newton’s method (frequently with an initial guess of 1), to compute the square root of a positive real number.[13][14] When computing square roots with logarithm tables or slide rules, one can exploit the identities
where ln and log10 are the natural and base-10 logarithms.
By trial-and-error,[15] one can square an estimate for 
as it allows one to adjust the estimate x by some amount c and measure the square of the adjustment in terms of the original estimate and its square. Furthermore, (x + c)2 ≈ x2 + 2xc when c is close to 0, because the tangent line to the graph of x2 + 2xc + c2 at c = 0, as a function of c alone, is y = 2xc + x2. Thus, small adjustments to x can be planned out by setting 2xc to a, or c = a/(2x).
The most common iterative method of square root calculation by hand is known as the «Babylonian method» or «Heron’s method» after the first-century Greek philosopher Heron of Alexandria, who first described it.[16]
The method uses the same iterative scheme as the Newton–Raphson method yields when applied to the function y = f(x) = x2 − a, using the fact that its slope at any point is dy/dx = f′(x) = 2x, but predates it by many centuries.[17]
The algorithm is to repeat a simple calculation that results in a number closer to the actual square root each time it is repeated with its result as the new input. The motivation is that if x is an overestimate to the square root of a nonnegative real number a then a/x will be an underestimate and so the average of these two numbers is a better approximation than either of them. However, the inequality of arithmetic and geometric means shows this average is always an overestimate of the square root (as noted below), and so it can serve as a new overestimate with which to repeat the process, which converges as a consequence of the successive overestimates and underestimates being closer to each other after each iteration. To find x:
- Start with an arbitrary positive start value x. The closer to the square root of a, the fewer the iterations that will be needed to achieve the desired precision.
- Replace x by the average (x + a/x) / 2 between x and a/x.
- Repeat from step 2, using this average as the new value of x.
That is, if an arbitrary guess for 

Using the identity
the computation of the square root of a positive number can be reduced to that of a number in the range [1,4). This simplifies finding a start value for the iterative method that is close to the square root, for which a polynomial or piecewise-linear approximation can be used.
The time complexity for computing a square root with n digits of precision is equivalent to that of multiplying two n-digit numbers.
Another useful method for calculating the square root is the shifting nth root algorithm, applied for n = 2.
The name of the square root function varies from programming language to programming language, with sqrt[18] (often pronounced «squirt» [19]) being common, used in C, C++, and derived languages like JavaScript, PHP, and Python.
Square roots of negative and complex numbers
First leaf of the complex square root
Second leaf of the complex square root
Using the Riemann surface of the square root, it is shown how the two leaves fit together
The square of any positive or negative number is positive, and the square of 0 is 0. Therefore, no negative number can have a real square root. However, it is possible to work with a more inclusive set of numbers, called the complex numbers, that does contain solutions to the square root of a negative number. This is done by introducing a new number, denoted by i (sometimes written as j, especially in the context of electricity where «i» traditionally represents electric current) and called the imaginary unit, which is defined such that i2 = −1. Using this notation, we can think of i as the square root of −1, but we also have (−i)2 = i2 = −1 and so −i is also a square root of −1. By convention, the principal square root of −1 is i, or more generally, if x is any nonnegative number, then the principal square root of −x is
The right side (as well as its negative) is indeed a square root of −x, since
For every non-zero complex number z there exist precisely two numbers w such that w2 = z: the principal square root of z (defined below), and its negative.
Principal square root of a complex number
Geometric representation of the 2nd to 6th roots of a complex number z, in polar form reiφ where r = |z | and φ = arg z. If z is real, φ = 0 or π. Principal roots are shown in black.
To find a definition for the square root that allows us to consistently choose a single value, called the principal value, we start by observing that any complex number 






then the principal square root of 
The principal square root function is thus defined using the nonpositive real axis as a branch cut.
If 



It is important that 


but using 
The principal square root function is holomorphic everywhere except on the set of non-positive real numbers (on strictly negative reals it is not even continuous). The above Taylor series for 


The above can also be expressed in terms of trigonometric functions:
Algebraic formula
When the number is expressed using its real and imaginary parts, the following formula can be used for the principal square root:[20][21]
where sgn(y) is the sign of y (except that, here, sgn(0) = 1). In particular, the imaginary parts of the original number and the principal value of its square root have the same sign. The real part of the principal value of the square root is always nonnegative.
For example, the principal square roots of ±i are given by:
Notes
In the following, the complex z and w may be expressed as:
where 

Because of the discontinuous nature of the square root function in the complex plane, the following laws are not true in general.
A similar problem appears with other complex functions with branch cuts, e.g., the complex logarithm and the relations logz + logw = log(zw) or log(z*) = log(z)* which are not true in general.
Wrongly assuming one of these laws underlies several faulty «proofs», for instance the following one showing that −1 = 1:
The third equality cannot be justified (see invalid proof).[22]: Chapter VI Some fallacies in algebra and trigonometry, Section I The fallacies, Subsection 2 The fallacy that +1 = -1 It can be made to hold by changing the meaning of √ so that this no longer represents the principal square root (see above) but selects a branch for the square root that contains 
if the branch includes +i or
if the branch includes −i, while the right-hand side becomes
where the last equality, 
Nth roots and polynomial roots
The definition of a square root of 


A cube root of 


![{displaystyle {sqrt[{3}]{x}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d19f445fd1e8ab7046f090279ee7cf3506f0cf50)
If n is an integer greater than two, a nth root of 


![{displaystyle {sqrt[{n}]{x}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8562e64a6bc6e408ddf67f055682c4dc9c9f957f)
Given any polynomial p, a root of p is a number y such that p(y) = 0. For example, the nth roots of x are the roots of the polynomial (in y) 
Abel–Ruffini theorem states that, in general, the roots of a polynomial of degree five or higher cannot be expressed in terms of nth roots.
Square roots of matrices and operators
If A is a positive-definite matrix or operator, then there exists precisely one positive definite matrix or operator B with B2 = A; we then define A1/2 = B. In general matrices may have multiple square roots or even an infinitude of them. For example, the 2 × 2 identity matrix has an infinity of square roots,[23] though only one of them is positive definite.
In integral domains, including fields
Each element of an integral domain has no more than 2 square roots. The difference of two squares identity u2 − v2 = (u − v)(u + v) is proved using the commutativity of multiplication. If u and v are square roots of the same element, then u2 − v2 = 0. Because there are no zero divisors this implies u = v or u + v = 0, where the latter means that two roots are additive inverses of each other. In other words if an element a square root u of an element a exists, then the only square roots of a are u and −u. The only square root of 0 in an integral domain is 0 itself.
In a field of characteristic 2, an element either has one square root or does not have any at all, because each element is its own additive inverse, so that −u = u. If the field is finite of characteristic 2 then every element has a unique square root. In a field of any other characteristic, any non-zero element either has two square roots, as explained above, or does not have any.
Given an odd prime number p, let q = pe for some positive integer e. A non-zero element of the field Fq with q elements is a quadratic residue if it has a square root in Fq. Otherwise, it is a quadratic non-residue. There are (q − 1)/2 quadratic residues and (q − 1)/2 quadratic non-residues; zero is not counted in either class. The quadratic residues form a group under multiplication. The properties of quadratic residues are widely used in number theory.
In rings in general
Unlike in an integral domain, a square root in an arbitrary (unital) ring need not be unique up to sign. For example, in the ring 
Another example is provided by the ring of quaternions 
A square root of 0 is either 0 or a zero divisor. Thus in rings where zero divisors do not exist, it is uniquely 0. However, rings with zero divisors may have multiple square roots of 0. For example, in 
Geometric construction of the square root
The square root of a positive number is usually defined as the side length of a square with the area equal to the given number. But the square shape is not necessary for it: if one of two similar planar Euclidean objects has the area a times greater than another, then the ratio of their linear sizes is 
A square root can be constructed with a compass and straightedge. In his Elements, Euclid (fl. 300 BC) gave the construction of the geometric mean of two quantities in two different places: Proposition II.14 and Proposition VI.13. Since the geometric mean of a and b is 

The construction is also given by Descartes in his La Géométrie, see figure 2 on page 2. However, Descartes made no claim to originality and his audience would have been quite familiar with Euclid.
Euclid’s second proof in Book VI depends on the theory of similar triangles. Let AHB be a line segment of length a + b with AH = a and HB = b. Construct the circle with AB as diameter and let C be one of the two intersections of the perpendicular chord at H with the circle and denote the length CH as h. Then, using Thales’ theorem and, as in the proof of Pythagoras’ theorem by similar triangles, triangle AHC is similar to triangle CHB (as indeed both are to triangle ACB, though we don’t need that, but it is the essence of the proof of Pythagoras’ theorem) so that AH:CH is as HC:HB, i.e. a/h = h/b, from which we conclude by cross-multiplication that h2 = ab, and finally that 

Another method of geometric construction uses right triangles and induction: 



See also
- Apotome (mathematics)
- Cube root
- Functional square root
- Integer square root
- Nested radical
- Nth root
- Root of unity
- Solving quadratic equations with continued fractions
- Square root principle
- Quantum gate § Square root of NOT gate (√NOT)
Notes
- ^ Gel’fand, p. 120 Archived 2016-09-02 at the Wayback Machine
- ^ «Squares and Square Roots». www.mathsisfun.com. Retrieved 2020-08-28.
- ^ Zill, Dennis G.; Shanahan, Patrick (2008). A First Course in Complex Analysis With Applications (2nd ed.). Jones & Bartlett Learning. p. 78. ISBN 978-0-7637-5772-4. Archived from the original on 2016-09-01. Extract of page 78 Archived 2016-09-01 at the Wayback Machine
- ^ Weisstein, Eric W. «Square Root». mathworld.wolfram.com. Retrieved 2020-08-28.
- ^ «Analysis of YBC 7289». ubc.ca. Retrieved 19 January 2015.
- ^ Anglin, W.S. (1994). Mathematics: A Concise History and Philosophy. New York: Springer-Verlag.
- ^ Joseph, ch.8.
- ^ Heath, Sir Thomas L. (1908). The Thirteen Books of The Elements, Vol. 3. Cambridge University Press. p. 3.
- ^ Dauben (2007), p. 210.
- ^ «The Development of Algebra — 2». maths.org. Archived from the original on 24 November 2014. Retrieved 19 January 2015.
- ^ * Oaks, Jeffrey A. (2012). Algebraic Symbolism in Medieval Arabic Algebra (PDF) (Thesis). Philosophica. p. 36. Archived (PDF) from the original on 2016-12-03.
- ^ Manguel, Alberto (2006). «Done on paper: the dual nature of numbers and the page». The Life of Numbers. ISBN 84-86882-14-1.
- ^ Parkhurst, David F. (2006). Introduction to Applied Mathematics for Environmental Science. Springer. pp. 241. ISBN 9780387342283.
- ^ Solow, Anita E. (1993). Learning by Discovery: A Lab Manual for Calculus. Cambridge University Press. pp. 48. ISBN 9780883850831.
- ^ Aitken, Mike; Broadhurst, Bill; Hladky, Stephen (2009). Mathematics for Biological Scientists. Garland Science. p. 41. ISBN 978-1-136-84393-8. Archived from the original on 2017-03-01. Extract of page 41 Archived 2017-03-01 at the Wayback Machine
- ^ Heath, Sir Thomas L. (1921). A History of Greek Mathematics, Vol. 2. Oxford: Clarendon Press. pp. 323–324.
- ^ Muller, Jean-Mic (2006). Elementary functions: algorithms and implementation. Springer. pp. 92–93. ISBN 0-8176-4372-9., Chapter 5, p 92 Archived 2016-09-01 at the Wayback Machine
- ^ «Function sqrt». CPlusPlus.com. The C++ Resources Network. 2016. Archived from the original on November 22, 2012. Retrieved June 24, 2016.
- ^ Overland, Brian (2013). C++ for the Impatient. Addison-Wesley. p. 338. ISBN 9780133257120. OCLC 850705706. Archived from the original on September 1, 2016. Retrieved June 24, 2016.
- ^ Abramowitz, Milton; Stegun, Irene A. (1964). Handbook of mathematical functions with formulas, graphs, and mathematical tables. Courier Dover Publications. p. 17. ISBN 0-486-61272-4. Archived from the original on 2016-04-23., Section 3.7.27, p. 17 Archived 2009-09-10 at the Wayback Machine
- ^ Cooke, Roger (2008). Classical algebra: its nature, origins, and uses. John Wiley and Sons. p. 59. ISBN 978-0-470-25952-8. Archived from the original on 2016-04-23.
- ^ Maxwell, E. A. (1959). Fallacies in Mathematics. Cambridge University Press.
- ^ Mitchell, Douglas W., «Using Pythagorean triples to generate square roots of I2«, Mathematical Gazette 87, November 2003, 499–500.
References
- Dauben, Joseph W. (2007). «Chinese Mathematics I». In Katz, Victor J. (ed.). The Mathematics of Egypt, Mesopotamia, China, India, and Islam. Princeton: Princeton University Press. ISBN 978-0-691-11485-9.
- Gel’fand, Izrael M.; Shen, Alexander (1993). Algebra (3rd ed.). Birkhäuser. p. 120. ISBN 0-8176-3677-3.
- Joseph, George (2000). The Crest of the Peacock. Princeton: Princeton University Press. ISBN 0-691-00659-8.
- Smith, David (1958). History of Mathematics. Vol. 2. New York: Dover Publications. ISBN 978-0-486-20430-7.
- Selin, Helaine (2008), Encyclopaedia of the History of Science, Technology, and Medicine in Non-Western Cultures, Springer, Bibcode:2008ehst.book…..S, ISBN 978-1-4020-4559-2.
External links
- Algorithms, implementations, and more – Paul Hsieh’s square roots webpage
- How to manually find a square root
- AMS Featured Column, Galileo’s Arithmetic by Tony Philips – includes a section on how Galileo found square roots
Notation for the (principal) square root of x.
For example, √25 = 5, since 25 = 5 ⋅ 5, or 52 (5 squared).
In mathematics, a square root of a number x is a number y such that y2 = x; in other words, a number y whose square (the result of multiplying the number by itself, or y ⋅ y) is x.[1] For example, 4 and −4 are square roots of 16, because 42 = (−4)2 = 16.
Every nonnegative real number x has a unique nonnegative square root, called the principal square root, which is denoted by 


Every positive number x has two square roots: 


Square roots of negative numbers can be discussed within the framework of complex numbers. More generally, square roots can be considered in any context in which a notion of the «square» of a mathematical object is defined. These include function spaces and square matrices, among other mathematical structures.
History
The Yale Babylonian Collection YBC 7289 clay tablet was created between 1800 BC and 1600 BC, showing 

The Rhind Mathematical Papyrus is a copy from 1650 BC of an earlier Berlin Papyrus and other texts – possibly the Kahun Papyrus – that shows how the Egyptians extracted square roots by an inverse proportion method.[6]
In Ancient India, the knowledge of theoretical and applied aspects of square and square root was at least as old as the Sulba Sutras, dated around 800–500 BC (possibly much earlier).[citation needed] A method for finding very good approximations to the square roots of 2 and 3 are given in the Baudhayana Sulba Sutra.[7] Aryabhata, in the Aryabhatiya (section 2.4), has given a method for finding the square root of numbers having many digits.
It was known to the ancient Greeks that square roots of positive integers that are not perfect squares are always irrational numbers: numbers not expressible as a ratio of two integers (that is, they cannot be written exactly as 
The particular case of the square root of 2 is assumed to date back earlier to the Pythagoreans, and is traditionally attributed to Hippasus.[citation needed] It is exactly the length of the diagonal of a square with side length 1.
In the Chinese mathematical work Writings on Reckoning, written between 202 BC and 186 BC during the early Han Dynasty, the square root is approximated by using an «excess and deficiency» method, which says to «…combine the excess and deficiency as the divisor; (taking) the deficiency numerator multiplied by the excess denominator and the excess numerator times the deficiency denominator, combine them as the dividend.»[9]
A symbol for square roots, written as an elaborate R, was invented by Regiomontanus (1436–1476). An R was also used for radix to indicate square roots in Gerolamo Cardano’s Ars Magna.[10]
According to historian of mathematics D.E. Smith, Aryabhata’s method for finding the square root was first introduced in Europe by Cataneo—in 1546.
According to Jeffrey A. Oaks, Arabs used the letter jīm/ĝīm (ج), the first letter of the word «جذر» (variously transliterated as jaḏr, jiḏr, ǧaḏr or ǧiḏr, «root»), placed in its initial form (ﺟ) over a number to indicate its square root. The letter jīm resembles the present square root shape. Its usage goes as far as the end of the twelfth century in the works of the Moroccan mathematician Ibn al-Yasamin.[11]
The symbol «√» for the square root was first used in print in 1525, in Christoph Rudolff’s Coss.[12]
Properties and uses
The graph of the function f(x) = √x, made up of half a parabola with a vertical directrix
The principal square root function 
The square root of x is rational if and only if x is a rational number that can be represented as a ratio of two perfect squares. (See square root of 2 for proofs that this is an irrational number, and quadratic irrational for a proof for all non-square natural numbers.) The square root function maps rational numbers into algebraic numbers, the latter being a superset of the rational numbers).
For all real numbers x,
(see absolute value)
For all nonnegative real numbers x and y,
and
The square root function is continuous for all nonnegative x, and differentiable for all positive x. If f denotes the square root function, whose derivative is given by:
The Taylor series of 
The square root of a nonnegative number is used in the definition of Euclidean norm (and distance), as well as in generalizations such as Hilbert spaces. It defines an important concept of standard deviation used in probability theory and statistics. It has a major use in the formula for roots of a quadratic equation; quadratic fields and rings of quadratic integers, which are based on square roots, are important in algebra and have uses in geometry. Square roots frequently appear in mathematical formulas elsewhere, as well as in many physical laws.
Square roots of positive integers
A positive number has two square roots, one positive, and one negative, which are opposite to each other. When talking of the square root of a positive integer, it is usually the positive square root that is meant.
The square roots of an integer are algebraic integers—more specifically quadratic integers.
The square root of a positive integer is the product of the roots of its prime factors, because the square root of a product is the product of the square roots of the factors. Since 
As decimal expansions
The square roots of the perfect squares (e.g., 0, 1, 4, 9, 16) are integers. In all other cases, the square roots of positive integers are irrational numbers, and hence have non-repeating decimals in their decimal representations. Decimal approximations of the square roots of the first few natural numbers are given in the following table.
-
n truncated to 50 decimal places
0 0 1 1 2 1.41421356237309504880168872420969807856967187537694 3 1.73205080756887729352744634150587236694280525381038 4 2 5 2.23606797749978969640917366873127623544061835961152 6 2.44948974278317809819728407470589139196594748065667 7 2.64575131106459059050161575363926042571025918308245 8 2.82842712474619009760337744841939615713934375075389 9 3 10 3.16227766016837933199889354443271853371955513932521
As expansions in other numeral systems
As with before, the square roots of the perfect squares (e.g., 0, 1, 4, 9, 16) are integers. In all other cases, the square roots of positive integers are irrational numbers, and therefore have non-repeating digits in any standard positional notation system.
The square roots of small integers are used in both the SHA-1 and SHA-2 hash function designs to provide nothing up my sleeve numbers.
As periodic continued fractions
One of the most intriguing results from the study of irrational numbers as continued fractions was obtained by Joseph Louis Lagrange c. 1780. Lagrange found that the representation of the square root of any non-square positive integer as a continued fraction is periodic. That is, a certain pattern of partial denominators repeats indefinitely in the continued fraction. In a sense these square roots are the very simplest irrational numbers, because they can be represented with a simple repeating pattern of integers.
-
= [1; 2, 2, …] = [1; 1, 2, 1, 2, …] = [2] = [2; 4, 4, …] = [2; 2, 4, 2, 4, …] = [2; 1, 1, 1, 4, 1, 1, 1, 4, …] = [2; 1, 4, 1, 4, …] = [3] = [3; 6, 6, …] = [3; 3, 6, 3, 6, …] = [3; 2, 6, 2, 6, …] = [3; 1, 1, 1, 1, 6, 1, 1, 1, 1, 6, …] = [3; 1, 2, 1, 6, 1, 2, 1, 6, …] = [3; 1, 6, 1, 6, …] = [4] = [4; 8, 8, …] = [4; 4, 8, 4, 8, …] = [4; 2, 1, 3, 1, 2, 8, 2, 1, 3, 1, 2, 8, …] = [4; 2, 8, 2, 8, …]
The square bracket notation used above is a short form for a continued fraction. Written in the more suggestive algebraic form, the simple continued fraction for the square root of 11, [3; 3, 6, 3, 6, …], looks like this:
where the two-digit pattern {3, 6} repeats over and over again in the partial denominators. Since 11 = 32 + 2, the above is also identical to the following generalized continued fractions:
Computation
Square roots of positive numbers are not in general rational numbers, and so cannot be written as a terminating or recurring decimal expression. Therefore in general any attempt to compute a square root expressed in decimal form can only yield an approximation, though a sequence of increasingly accurate approximations can be obtained.
Most pocket calculators have a square root key. Computer spreadsheets and other software are also frequently used to calculate square roots. Pocket calculators typically implement efficient routines, such as the Newton’s method (frequently with an initial guess of 1), to compute the square root of a positive real number.[13][14] When computing square roots with logarithm tables or slide rules, one can exploit the identities
where ln and log10 are the natural and base-10 logarithms.
By trial-and-error,[15] one can square an estimate for 
as it allows one to adjust the estimate x by some amount c and measure the square of the adjustment in terms of the original estimate and its square. Furthermore, (x + c)2 ≈ x2 + 2xc when c is close to 0, because the tangent line to the graph of x2 + 2xc + c2 at c = 0, as a function of c alone, is y = 2xc + x2. Thus, small adjustments to x can be planned out by setting 2xc to a, or c = a/(2x).
The most common iterative method of square root calculation by hand is known as the «Babylonian method» or «Heron’s method» after the first-century Greek philosopher Heron of Alexandria, who first described it.[16]
The method uses the same iterative scheme as the Newton–Raphson method yields when applied to the function y = f(x) = x2 − a, using the fact that its slope at any point is dy/dx = f′(x) = 2x, but predates it by many centuries.[17]
The algorithm is to repeat a simple calculation that results in a number closer to the actual square root each time it is repeated with its result as the new input. The motivation is that if x is an overestimate to the square root of a nonnegative real number a then a/x will be an underestimate and so the average of these two numbers is a better approximation than either of them. However, the inequality of arithmetic and geometric means shows this average is always an overestimate of the square root (as noted below), and so it can serve as a new overestimate with which to repeat the process, which converges as a consequence of the successive overestimates and underestimates being closer to each other after each iteration. To find x:
- Start with an arbitrary positive start value x. The closer to the square root of a, the fewer the iterations that will be needed to achieve the desired precision.
- Replace x by the average (x + a/x) / 2 between x and a/x.
- Repeat from step 2, using this average as the new value of x.
That is, if an arbitrary guess for 

Using the identity
the computation of the square root of a positive number can be reduced to that of a number in the range [1,4). This simplifies finding a start value for the iterative method that is close to the square root, for which a polynomial or piecewise-linear approximation can be used.
The time complexity for computing a square root with n digits of precision is equivalent to that of multiplying two n-digit numbers.
Another useful method for calculating the square root is the shifting nth root algorithm, applied for n = 2.
The name of the square root function varies from programming language to programming language, with sqrt[18] (often pronounced «squirt» [19]) being common, used in C, C++, and derived languages like JavaScript, PHP, and Python.
Square roots of negative and complex numbers
First leaf of the complex square root
Second leaf of the complex square root
Using the Riemann surface of the square root, it is shown how the two leaves fit together
The square of any positive or negative number is positive, and the square of 0 is 0. Therefore, no negative number can have a real square root. However, it is possible to work with a more inclusive set of numbers, called the complex numbers, that does contain solutions to the square root of a negative number. This is done by introducing a new number, denoted by i (sometimes written as j, especially in the context of electricity where «i» traditionally represents electric current) and called the imaginary unit, which is defined such that i2 = −1. Using this notation, we can think of i as the square root of −1, but we also have (−i)2 = i2 = −1 and so −i is also a square root of −1. By convention, the principal square root of −1 is i, or more generally, if x is any nonnegative number, then the principal square root of −x is
The right side (as well as its negative) is indeed a square root of −x, since
For every non-zero complex number z there exist precisely two numbers w such that w2 = z: the principal square root of z (defined below), and its negative.
Principal square root of a complex number
Geometric representation of the 2nd to 6th roots of a complex number z, in polar form reiφ where r = |z | and φ = arg z. If z is real, φ = 0 or π. Principal roots are shown in black.
To find a definition for the square root that allows us to consistently choose a single value, called the principal value, we start by observing that any complex number 






then the principal square root of 
The principal square root function is thus defined using the nonpositive real axis as a branch cut.
If 



It is important that 


but using 
The principal square root function is holomorphic everywhere except on the set of non-positive real numbers (on strictly negative reals it is not even continuous). The above Taylor series for 


The above can also be expressed in terms of trigonometric functions:
Algebraic formula
When the number is expressed using its real and imaginary parts, the following formula can be used for the principal square root:[20][21]
where sgn(y) is the sign of y (except that, here, sgn(0) = 1). In particular, the imaginary parts of the original number and the principal value of its square root have the same sign. The real part of the principal value of the square root is always nonnegative.
For example, the principal square roots of ±i are given by:
Notes
In the following, the complex z and w may be expressed as:
where 

Because of the discontinuous nature of the square root function in the complex plane, the following laws are not true in general.
A similar problem appears with other complex functions with branch cuts, e.g., the complex logarithm and the relations logz + logw = log(zw) or log(z*) = log(z)* which are not true in general.
Wrongly assuming one of these laws underlies several faulty «proofs», for instance the following one showing that −1 = 1:
The third equality cannot be justified (see invalid proof).[22]: Chapter VI Some fallacies in algebra and trigonometry, Section I The fallacies, Subsection 2 The fallacy that +1 = -1 It can be made to hold by changing the meaning of √ so that this no longer represents the principal square root (see above) but selects a branch for the square root that contains 
if the branch includes +i or
if the branch includes −i, while the right-hand side becomes
where the last equality, 
Nth roots and polynomial roots
The definition of a square root of 


A cube root of 


![{displaystyle {sqrt[{3}]{x}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/d19f445fd1e8ab7046f090279ee7cf3506f0cf50)
If n is an integer greater than two, a nth root of 


![{displaystyle {sqrt[{n}]{x}}.}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8562e64a6bc6e408ddf67f055682c4dc9c9f957f)
Given any polynomial p, a root of p is a number y such that p(y) = 0. For example, the nth roots of x are the roots of the polynomial (in y) 
Abel–Ruffini theorem states that, in general, the roots of a polynomial of degree five or higher cannot be expressed in terms of nth roots.
Square roots of matrices and operators
If A is a positive-definite matrix or operator, then there exists precisely one positive definite matrix or operator B with B2 = A; we then define A1/2 = B. In general matrices may have multiple square roots or even an infinitude of them. For example, the 2 × 2 identity matrix has an infinity of square roots,[23] though only one of them is positive definite.
In integral domains, including fields
Each element of an integral domain has no more than 2 square roots. The difference of two squares identity u2 − v2 = (u − v)(u + v) is proved using the commutativity of multiplication. If u and v are square roots of the same element, then u2 − v2 = 0. Because there are no zero divisors this implies u = v or u + v = 0, where the latter means that two roots are additive inverses of each other. In other words if an element a square root u of an element a exists, then the only square roots of a are u and −u. The only square root of 0 in an integral domain is 0 itself.
In a field of characteristic 2, an element either has one square root or does not have any at all, because each element is its own additive inverse, so that −u = u. If the field is finite of characteristic 2 then every element has a unique square root. In a field of any other characteristic, any non-zero element either has two square roots, as explained above, or does not have any.
Given an odd prime number p, let q = pe for some positive integer e. A non-zero element of the field Fq with q elements is a quadratic residue if it has a square root in Fq. Otherwise, it is a quadratic non-residue. There are (q − 1)/2 quadratic residues and (q − 1)/2 quadratic non-residues; zero is not counted in either class. The quadratic residues form a group under multiplication. The properties of quadratic residues are widely used in number theory.
In rings in general
Unlike in an integral domain, a square root in an arbitrary (unital) ring need not be unique up to sign. For example, in the ring 
Another example is provided by the ring of quaternions 
A square root of 0 is either 0 or a zero divisor. Thus in rings where zero divisors do not exist, it is uniquely 0. However, rings with zero divisors may have multiple square roots of 0. For example, in 
Geometric construction of the square root
The square root of a positive number is usually defined as the side length of a square with the area equal to the given number. But the square shape is not necessary for it: if one of two similar planar Euclidean objects has the area a times greater than another, then the ratio of their linear sizes is 
A square root can be constructed with a compass and straightedge. In his Elements, Euclid (fl. 300 BC) gave the construction of the geometric mean of two quantities in two different places: Proposition II.14 and Proposition VI.13. Since the geometric mean of a and b is 

The construction is also given by Descartes in his La Géométrie, see figure 2 on page 2. However, Descartes made no claim to originality and his audience would have been quite familiar with Euclid.
Euclid’s second proof in Book VI depends on the theory of similar triangles. Let AHB be a line segment of length a + b with AH = a and HB = b. Construct the circle with AB as diameter and let C be one of the two intersections of the perpendicular chord at H with the circle and denote the length CH as h. Then, using Thales’ theorem and, as in the proof of Pythagoras’ theorem by similar triangles, triangle AHC is similar to triangle CHB (as indeed both are to triangle ACB, though we don’t need that, but it is the essence of the proof of Pythagoras’ theorem) so that AH:CH is as HC:HB, i.e. a/h = h/b, from which we conclude by cross-multiplication that h2 = ab, and finally that 

Another method of geometric construction uses right triangles and induction: 



See also
- Apotome (mathematics)
- Cube root
- Functional square root
- Integer square root
- Nested radical
- Nth root
- Root of unity
- Solving quadratic equations with continued fractions
- Square root principle
- Quantum gate § Square root of NOT gate (√NOT)
Notes
- ^ Gel’fand, p. 120 Archived 2016-09-02 at the Wayback Machine
- ^ «Squares and Square Roots». www.mathsisfun.com. Retrieved 2020-08-28.
- ^ Zill, Dennis G.; Shanahan, Patrick (2008). A First Course in Complex Analysis With Applications (2nd ed.). Jones & Bartlett Learning. p. 78. ISBN 978-0-7637-5772-4. Archived from the original on 2016-09-01. Extract of page 78 Archived 2016-09-01 at the Wayback Machine
- ^ Weisstein, Eric W. «Square Root». mathworld.wolfram.com. Retrieved 2020-08-28.
- ^ «Analysis of YBC 7289». ubc.ca. Retrieved 19 January 2015.
- ^ Anglin, W.S. (1994). Mathematics: A Concise History and Philosophy. New York: Springer-Verlag.
- ^ Joseph, ch.8.
- ^ Heath, Sir Thomas L. (1908). The Thirteen Books of The Elements, Vol. 3. Cambridge University Press. p. 3.
- ^ Dauben (2007), p. 210.
- ^ «The Development of Algebra — 2». maths.org. Archived from the original on 24 November 2014. Retrieved 19 January 2015.
- ^ * Oaks, Jeffrey A. (2012). Algebraic Symbolism in Medieval Arabic Algebra (PDF) (Thesis). Philosophica. p. 36. Archived (PDF) from the original on 2016-12-03.
- ^ Manguel, Alberto (2006). «Done on paper: the dual nature of numbers and the page». The Life of Numbers. ISBN 84-86882-14-1.
- ^ Parkhurst, David F. (2006). Introduction to Applied Mathematics for Environmental Science. Springer. pp. 241. ISBN 9780387342283.
- ^ Solow, Anita E. (1993). Learning by Discovery: A Lab Manual for Calculus. Cambridge University Press. pp. 48. ISBN 9780883850831.
- ^ Aitken, Mike; Broadhurst, Bill; Hladky, Stephen (2009). Mathematics for Biological Scientists. Garland Science. p. 41. ISBN 978-1-136-84393-8. Archived from the original on 2017-03-01. Extract of page 41 Archived 2017-03-01 at the Wayback Machine
- ^ Heath, Sir Thomas L. (1921). A History of Greek Mathematics, Vol. 2. Oxford: Clarendon Press. pp. 323–324.
- ^ Muller, Jean-Mic (2006). Elementary functions: algorithms and implementation. Springer. pp. 92–93. ISBN 0-8176-4372-9., Chapter 5, p 92 Archived 2016-09-01 at the Wayback Machine
- ^ «Function sqrt». CPlusPlus.com. The C++ Resources Network. 2016. Archived from the original on November 22, 2012. Retrieved June 24, 2016.
- ^ Overland, Brian (2013). C++ for the Impatient. Addison-Wesley. p. 338. ISBN 9780133257120. OCLC 850705706. Archived from the original on September 1, 2016. Retrieved June 24, 2016.
- ^ Abramowitz, Milton; Stegun, Irene A. (1964). Handbook of mathematical functions with formulas, graphs, and mathematical tables. Courier Dover Publications. p. 17. ISBN 0-486-61272-4. Archived from the original on 2016-04-23., Section 3.7.27, p. 17 Archived 2009-09-10 at the Wayback Machine
- ^ Cooke, Roger (2008). Classical algebra: its nature, origins, and uses. John Wiley and Sons. p. 59. ISBN 978-0-470-25952-8. Archived from the original on 2016-04-23.
- ^ Maxwell, E. A. (1959). Fallacies in Mathematics. Cambridge University Press.
- ^ Mitchell, Douglas W., «Using Pythagorean triples to generate square roots of I2«, Mathematical Gazette 87, November 2003, 499–500.
References
- Dauben, Joseph W. (2007). «Chinese Mathematics I». In Katz, Victor J. (ed.). The Mathematics of Egypt, Mesopotamia, China, India, and Islam. Princeton: Princeton University Press. ISBN 978-0-691-11485-9.
- Gel’fand, Izrael M.; Shen, Alexander (1993). Algebra (3rd ed.). Birkhäuser. p. 120. ISBN 0-8176-3677-3.
- Joseph, George (2000). The Crest of the Peacock. Princeton: Princeton University Press. ISBN 0-691-00659-8.
- Smith, David (1958). History of Mathematics. Vol. 2. New York: Dover Publications. ISBN 978-0-486-20430-7.
- Selin, Helaine (2008), Encyclopaedia of the History of Science, Technology, and Medicine in Non-Western Cultures, Springer, Bibcode:2008ehst.book…..S, ISBN 978-1-4020-4559-2.
External links
- Algorithms, implementations, and more – Paul Hsieh’s square roots webpage
- How to manually find a square root
- AMS Featured Column, Galileo’s Arithmetic by Tony Philips – includes a section on how Galileo found square roots
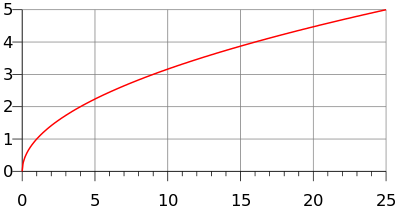
√ Квадратный корень
Нажмите, чтобы скопировать и вставить символ
Значение символа
Математический знак корня (√) обозначает операцию обратную возведению в степень. На клавиатуре (в Windows) для него есть специальная комбинация клавиш: Alt+251. Набирать нужно на цифровом блоке с включённым Num Lock. Также, можно скопировать отсюда.
По умолчанию, корень является квадратным, то есть обратен операции возведения в квадрат. Степень задаётся маленькой цифрой перед символом. В Юникоде также присутствуют знаки корней третьей (кубический) и четвёртой степеней ∛, ∜. Есть ещё арабские ؆, но я их вам не предлагаю. Извлечение корня можно ещё записать как возведение в дробную степень: ∜(x) = (x)^0.25.
В средние века вместо знака корня применяли латинскую заглавную R, как сокращение латинского слова Radix, что значит корень. Позже стали использовать стилизованную маленькую r, которая уже была очень похожа на современный значок. Первым это сделал Кристоф Рудольф в 1525 году. Черта корня над выражением появилась ещё позже. Сначала, вместо скобок, её использовал Декарт в 1637 году. Позже она слилась со знаком корня.
Символ «Квадратный корень» был утвержден как часть Юникода версии 1.1 в 1993 г.
Свойства
| Версия | 1.1 |
| Блок | Математические операторы |
| Тип парной зеркальной скобки (bidi) | Нет |
| Композиционное исключение | Нет |
| Изменение регистра | 221A |
| Простое изменение регистра | 221A |
Похожие символы
-
Жирная незакрашенная отметка галочка
-
𝥁
Жестовая письменность вертикальное…
-
☒
Избирательная урна с x
-
✘
Жирный крестик в анкете голосования
-
✗
Крестик в анкете голосования
-
Жирный знак умножения
-
Белый крест в квадрате
-
Метка крест
-
𝥀
Жестовая письменность вертикальное…
-
✓
Символ галочка
-
𝤿
Жестовая письменность вертикальное…
-
𝥷
Жестовая письменность горизонтальное…
-
⍻
Знак не проверено
-
🗸
Тонкая отметка «галочка»
-
Избирательная урна с галочкой
Кодировка
| Кодировка | hex | dec (bytes) | dec | binary |
|---|---|---|---|---|
| UTF-8 | E2 88 9A | 226 136 154 | 14846106 | 11100010 10001000 10011010 |
| UTF-16BE | 22 1A | 34 26 | 8730 | 00100010 00011010 |
| UTF-16LE | 1A 22 | 26 34 | 6690 | 00011010 00100010 |
| UTF-32BE | 00 00 22 1A | 0 0 34 26 | 8730 | 00000000 00000000 00100010 00011010 |
| UTF-32LE | 1A 22 00 00 | 26 34 0 0 | 438435840 | 00011010 00100010 00000000 00000000 |
У ряда пользователей, активно работающих с математикой, статистикой и прочими точными науками может возникнуть потребность набрать на клавиатуре символ корня √. При этом ни на одной из кнопок клавиатуры нет изображения подобного символа, и пользователь задаётся вопросом: как же осуществить подобное? В этом материале я помогу таким пользователям расскажу о вводе корня на клавиатуре, поясню, какие методы для этого существуют, и как обозначить корни 3,4,5 степеней.
Содержание
- Как поставить знак квадратный корень на клавиатуре
- Как использовать таблицу символов
- Как обозначить степени корня 3,4,5 степени на клавиатуре
- Заключение
Как поставить знак квадратный корень на клавиатуре
Многие пользователи в решении вопроса о написании корня на ПК, используют суррогатный символ «^», расположенный на клавише 6 в верхней части клавиатуры (активируется переходом на английскую раскладку, нажатием клавиши Shift и кнопки «6» сверху).
Некоторые пользователи также пользуются буквосочетанием sqrt (для квадратных корней), cbrt (для кубических) и так далее.
При этом это хоть и быстрые, но недостаточные приёмы. Для нормального набора знака корня выполните следующее:
- Нажмите кнопку Num Lock (должен зажечься соответствующий индикатор);
- Нажмите и не отжимайте кнопку Alt;
- Наберите на цифровой клавиатуре справа 251 и отожмите клавишу;
- Вы получите изображение квадратного корня √.
Если вы не знаете, как ввести собаку с клавиатуры, тогда вам обязательно нужно ознакомить с подробной инструкцией по её вводу, так как при наборе E-mail почты без знака собачки не обойтись.
Как использовать таблицу символов
Альтернативой этому варианту является использование специальной таблицы символов, имеющейся в ОС Виндовс, позволяющей использовать корень на клавиатуре. Выполните следующее:
- Нажмите на «Пуск», затем выберите «Все программы»;
- Потом «Стандартные», затем «Служебные», где выберите «Таблица символов».
- Там найдите знак корня √, кликните на него, нажмите на кнопку «Выбрать», затем «Копировать» и скопируйте его в нужный вам текст с помощью клавиш Ctrl+V.
В текстовом редакторе Word (а также в Excel) также имеется соответствующая таблица символов, которую можно использовать для наших задач. Вы можете найти её, перейдя во вкладку «Вставка», и нажав на «Символ» справа, а затем и кликнув на надпись «Другие символы» чуть снизу, это поможет вам в решении вопроса написании корня в Ворде.
Можно, также, использовать опцию «Формула» во вкладке «Вставка» по описанному в данном ролике алгоритму.
Как обозначить степени корня 3,4,5 степени на клавиатуре
Например, корни 3,4,5 степени можно записать так:
X^1/3
X^1/4
X^1/5
Или так:
3√X (вместо числа 3 можете использовать соответствующее обозначение из таблицы символов (³)
4√X
5√X
При этом, несмотря на то, что в системе имеется изображение кубического корня ∛ и четвёртого корня ∜, набрать их через Alt и цифровые клавиши не получится. Это возможно лишь с помощью кодов десятичной системы HTML-код (∛ и ∜) и шестнадцатеричной Юникод (∛ и ∜). По мне, так лучше использовать формы обозначения, описанные мной чуть выше.
Заключение
В данном материале мной были описаны разные варианты написания корня на клавиатуре вашего компьютера. Самые нетерпеливые могут воспользоваться знаком ^, но точнее и правильнее будет, всё же, воспользоваться комбинацией клавиш Alt+251, и поставить знак корня таким, каким он обозначается в соответствии с общепризнанным стандартом символов.
5 способов поставить знак корня на компьютере в ворде и других программах, на телефоне и планшете
Просмотров 53.9к. Опубликовано 29.06.2020 Обновлено 04.10.2021
Есть символы, которые используются редко и, кажется, что на компьютере их поставить невозможно. Проще распечатать документ, вписать их вручную, или использовать какое-то буквенное обозначение. Среди таких элементов – квадратный корень √. Найти значок корня на клавиатуре невозможно. Но это не означает, что ставить его нельзя. Читайте нашу статью, в ней мы готовы показать вам, как любую математическую, физическую запись сделать изящной и красивой на пк, ноутбуке, телефоне.
Содержание
- Где в компьютере используется квадратный корень √
- Способы набора корня на клавиатуре компьютера и ноутбука
- Используем таблицу символов
- Код символа
- Значок корня в уравнении
- Шестнадцатеричный код
- Способы набора символа в ворде
- На телефоне
- Проблемы со вставкой символа корня
- Выводы
Где в компьютере используется квадратный корень √
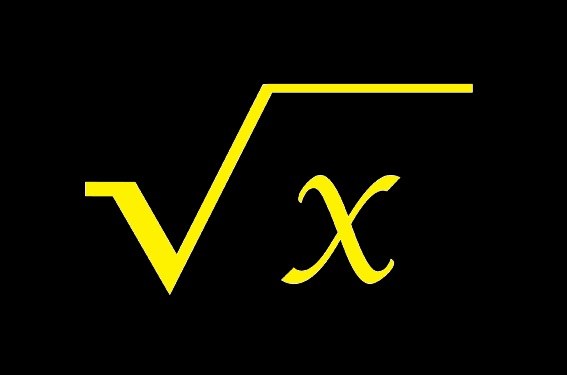
Среди таких работ:
- дипломы по математике;
- курсовые по алгебре, информатике;
- инженерные расчеты;
- запись результатов исследований по физике:
- калькуляции строительных, ремонтных работ;
- научные исследования.
Способы набора корня на клавиатуре компьютера и ноутбука
Часто, чтобы поставить в текст радикал или запись с ним, используются сочетания букв. Sqrt, например, означает кв. корень, а cbrt – кубический. Но писать буквенные комбинации, слова неудобно. Кроме того, они не всем понятны.
Удобнее использовать известный элемент √, похожий на галочку с одной длинной стороной. Способов вставить в текст его не так много. О каждом мы сейчас подробно расскажем.
Используем таблицу символов
Что такое арифметический квадратный корень
Квадратным корнем (арифметическим квадратным корнем) из неотрицательного числа (a) называется такое неотрицательное число, квадрат которого равен (a). ( (sqrt{a}=x, {{x}^{2}}=a; x, age 0)).
А почему же число ( a) (число под корнем) должно быть обязательно неотрицательным?
Например, чему равен ( sqrt{-9})?
Так-так, попробуем подобрать. Может, три?
Проверим: ( {{3}^{2}}=9), а не ( -9).
Может, ( left( -3 right))?
Опять же, проверяем: ( {{left( -3 right)}^{2}}=9).
Ну что же, не подбирается?
Это и следовало ожидать – потому что нет таких чисел, которые при возведении в квадрат дают отрицательное число! Это надо запомнить!
Число или выражение под знаком корня должно быть неотрицательным!
Однако ты наверняка уже заметил, что не только число под корнем должно быть неотрицательным, но и само значение тоже должно быть неотрицательным!
Ведь в определении сказано, что «квадратным корнем из числа( a)называется такое неотрицательное число, квадрат которого равен( a)».
Но подождите! В самом начале мы разбирали пример ( {{x}^{2}}=4) и один из ответов был отрицательным числом!
Мы подбирали числа, которые можно возвести в квадрат и получить при этом ( displaystyle 4). Ответом были ( displaystyle 2) и ( displaystyle -2)
А тут говорится, что квадратным корнем должно быть «неотрицательное число»! Почему?
Такой вопрос вполне уместен. Здесь необходимо просто разграничить понятия квадратного уравнения и арифметического квадратного корня.
К примеру, ( displaystyle {{x}^{2}}=4) (квадратное уравнение) не равносильно выражению ( x=sqrt{4}) (арифмитический квадратный корень).
Из ( {{x}^{2}}=4) следует, что
( left| x right|=sqrt{4}), то есть ( x=pm sqrt{4}=pm 2) или ( {{x}_{1}}=2); ( {{x}_{2}}=-2)
(не помнишь почему так? Почитай тему “Модуль числа”!)
А из ( x=sqrt{4}) следует, что ( x=2).
Конечно, это очень путает, но это необходимо запомнить, что знаки “плюс-минус” являются результатом решения квадратного уравнения, так как при решении уравнения мы должны записать все иксы, которые при подстановке в исходное уравнение дадут верный результат.
В наше квадратное уравнение подходит как ( 2), так и ( x=-2).
Однако, если просто извлекать квадратный корень из чего-либо, то всегда получаем один неотрицательный результат.
Запись иррациональных чисел с помощью квадратного корня
А теперь попробуй решить такое уравнение ( {{x}^{2}}=3).
Уже все не так просто и гладко, правда? Попробуй перебрать числа, может, что-то и выгорит?
Начнем с самого начала – с нуля: ( {{0}^{2}}=0) – не подходит.
Двигаемся дальше ( displaystyle x=1); ( displaystyle {{1}^{2}}=1) – меньше трех, тоже отметаем.
А что если ( displaystyle x=2)?
Проверим: ( displaystyle {{2}^{2}}=4) – тоже не подходит, т.к. это больше трех.
С отрицательными числами получится такая же история.
И что же теперь делать? Неужели перебор нам ничего не дал?
Совсем нет, теперь мы точно знаем, что ответом будет некоторое число между ( displaystyle 1) и ( displaystyle 2), а также между ( displaystyle -2) и ( displaystyle -1).
Кроме того, очевидно, что решения не будут целыми числами. Более того, они не являются рациональными.
И что дальше?
Давай построим график функции ( displaystyle y={{x}^{2}}) и отметим на нем решения.
Попробуем обмануть систему и получить ответ с помощью калькулятора (как мы это делали в начале)!
Извлечем корень из ( displaystyle 3), делов-то!
Ой-ой-ой, выходит, что ( sqrt{3}=1,732050807568ldots ) Такое число никогда не кончается.
Как же такое запомнить, ведь на экзамене калькулятора не будет!?
Все очень просто, это и не надо запоминать, необходимо помнить (или уметь быстро прикинуть) приблизительное значение. ( sqrt{3}) и ( -sqrt{3}) уже сами по себе ответы.
Такие числа называются иррациональными, именно для упрощения записи таких чисел и было введено понятие квадратного корня.
Деление корней
С умножением корней разобрались, теперь приступим к свойству деления.
Напомню, что формула в общем виде выглядит так:
( displaystyle sqrt[{}]{frac{a}{b}}=frac{sqrt[{}]{a}}{sqrt[{}]{b}}), если ( displaystyle age 0 , b>0).
А значит это, что корень из частного равен частному корней.
Ну что, давай разбираться на примерах:
( displaystyle frac{sqrt{12}}{sqrt{3}}=sqrt{frac{12}{3}}=sqrt{4}=2)
Вот и вся наука. А вот такой пример:
( displaystyle frac{sqrt{12}}{3}=frac{sqrt{12}}{sqrt{9}}=sqrt{frac{12}{9}}=sqrt{frac{4}{3}}=frac{2}{sqrt{3}})
Все не так гладко, как в первом примере, но, как видишь, ничего сложного нет.
А что, если попадется такое выражение:
( displaystyle sqrt{frac{144}{225}}=?)
Надо просто применить формулу в обратном направлении:
( displaystyle sqrt{frac{144}{225}}=frac{sqrt{144}}{sqrt{225}}=frac{12}{15}=frac{4}{5}=0,8)
А вот такой примерчик:
( displaystyle sqrt{0,16}=sqrt{frac{16}{100}}=frac{4}{10}=0,4)
Еще ты можешь встретить такое выражение:
( displaystyle sqrt{5frac{19}{25}}=?)
Все то же самое, только здесь надо вспомнить, как переводить дроби (если не помнишь, загляни в тему дроби и возвращайся!). Вспомнил? Теперь решаем!
( displaystyle sqrt{5frac{19}{25}}=sqrt{frac{144}{25}}=frac{12}{5}=2,4)
Уверена, что ты со всем, всем справился, теперь попробуем возводить корни в степени.
Возведение в степень
А что же будет, если квадратный корень возвести в квадрат? Все просто, вспомним смысл квадратного корня из числа ( displaystyle a) – это число, квадратный корень которого равен ( displaystyle a).
Так вот, если мы возводим число, квадратный корень которого равен ( displaystyle a), в квадрат, то что получаем?
Ну, конечно, ( displaystyle a)!
Рассмотрим на примерах:
( displaystyle {{left( sqrt{12} right)}^{2}}=12)
( displaystyle {{left( sqrt{17} right)}^{2}}=17)
Все просто, правда? А если корень будет в другой степени? Ничего страшного!
Придерживайся той же логики и помни свойства и возможные действия со степенями.
Забыл?
Почитай теорию по теме «Степень и ее свойства» и тебе все станет предельно ясно.
Вот, к примеру, такое выражение:
( displaystyle {{left( sqrt{5} right)}^{6}}={{left( {{left( sqrt{5} right)}^{2}} right)}^{3}}={{5}^{3}}=125)
В этом примере степень четная, а если она будет нечетная? Опять же, примени свойства степени и разложи все на множители:
( displaystyle {{left( sqrt{5} right)}^{7}}={{left( sqrt{5} right)}^{6}}cdot sqrt{5}=125sqrt{5})
С этим вроде все ясно, а как извлечь корень из числа в степени? Вот, к примеру, такое:
( displaystyle sqrt{{{3}^{2}}}=sqrt{9}=3)
Довольно просто, правда? А если степень больше двух? Следуем той же логике, используя свойства степеней:
( displaystyle sqrt{{{3}^{6}}}=sqrt{{{left( {{3}^{3}} right)}^{2}}}={{3}^{3}}=27)
( displaystyle sqrt{{{3}^{5}}}=sqrt{{{3}^{4}}cdot 3}=sqrt{{{left( {{3}^{2}} right)}^{2}}cdot 3}={{3}^{2}}cdot sqrt{3}=9sqrt{3})
Ну как, все понятно? Тогда реши самостоятельно примеры:
- ( displaystyle sqrt{{{left( -3 right)}^{2}}})
- ( displaystyle sqrt{{{6}^{6}}})
- ( displaystyle {{left( sqrt{8} right)}^{7}})
А вот и ответы:
Внесение под знак корня
Что мы только не научились делать с корнями! Осталось только потренироваться вносить число под знак корня!
Это совсем легко!
( displaystyle 4sqrt{6}-2sqrt{3}cdot sqrt{8}=sqrt{16cdot 6}-sqrt{4cdot 3cdot 8}=sqrt{96}-sqrt{96}=0)
Допустим, у нас записано число ( displaystyle 3sqrt{5})
Что мы можем с ним сделать? Ну конечно, спрятать тройку под корнем, помня при этом, что тройка – корень квадратный из ( displaystyle 9)!
( displaystyle 3sqrt{5}=sqrt{9}cdot sqrt{5}=sqrt{45})
Зачем нам это нужно? Да просто, чтобы расширить наши возможности при решении примеров:
( displaystyle 3sqrt{10}-sqrt{45}cdot sqrt{2}=sqrt{90}-sqrt{90}=0)
Как тебе такое свойство корней? Существенно упрощает жизнь? По мне, так точно! Только надо помнить, что вносить под знак квадратного корня мы можем только положительные числа.
Реши самостоятельно вот этот пример – ( displaystyle 4sqrt{6}-2sqrt{3}cdot sqrt{8})
Справился? Давай смотреть, что у тебя должно получиться:
( displaystyle 4sqrt{6}-2sqrt{3}cdot sqrt{8}=sqrt{16cdot 6}-sqrt{4cdot 3cdot 8}=sqrt{96}-sqrt{96}=0)
Молодец! У тебя получилось внести число под знак корня! Перейдем к не менее важному – рассмотрим, как сравнивать числа, содержащие квадратный корень!
Сравнение корней
Зачем нам учиться сравнивать числа, содержащие квадратный корень?
Очень просто. Часто, в больших и длиииинных выражениях, встречающихся на экзамене, мы получаем иррациональный ответ (помнишь, что это такое? Мы с тобой сегодня об этом уже говорили!)
Полученные ответы нам необходимо расположить на координатной прямой, например, чтобы определить, какой интервал подходит для решения уравнения. И вот здесь возникает загвоздка: калькулятора на экзамене нет, а без него как представить какое число больше, а какое меньше? То-то и оно!
Например, определи, что больше: ( displaystyle 3sqrt{7}) или ( displaystyle 2sqrt{17})?
Сходу и не скажешь. Ну что, воспользуемся разобранным свойством внесения числа под знак корня?
Тогда вперед:
( displaystyle 3sqrt{7}=sqrt{9cdot 7}=sqrt{63})
( displaystyle 2sqrt{17}=sqrt{4cdot 17}=sqrt{68})
Ну и, очевидно, что чем больше число под знаком корня, тем больше сам корень!
Т.е. если ( displaystyle 68>63), значит, ( displaystyle sqrt{68}>sqrt{63}).
Отсюда твердо делаем вывод, что ( displaystyle 3sqrt{7}<2sqrt{17}).
И никто не убедит нас в обратном!
Извлечение корней из больших чисел
До этого мы вносили множитель под знак корня, а как его вынести? Надо просто разложить его на множители и извлечь то, что извлекается!
( displaystyle sqrt{98}=sqrt{49cdot 2}=sqrt{49}cdot sqrt{2}=7sqrt{2})
Можно было пойти по иному пути и разложить на другие множители:
( displaystyle sqrt{98}=sqrt{7cdot 14})
Что дальше? А дальше раскладываем на множители до самого конца:
( displaystyle sqrt{98}=sqrt{7cdot 14}=sqrt{7cdot 7cdot 2}=sqrt{{{7}^{2}}cdot 2}=7sqrt{2})
Неплохо, да? Любой из этих подходов верен, решай как тебе удобно.
Разложение на множители очень пригодится при решении таких нестандартных заданий, как вот это:
( displaystyle sqrt{15}cdot sqrt{180}cdot sqrt{12})
Не пугаемся, а действуем! Разложим каждый множитель под корнем на отдельные множители:
А теперь попробуй самостоятельно (без калькулятора! его на экзамене не будет):
( displaystyle sqrt{15}cdot sqrt{180}cdot sqrt{12}=sqrt{5cdot 3}cdot sqrt{36cdot 5}cdot sqrt{2cdot 6})
Разве это конец? Не останавливаемся на полпути!
( displaystyle begin{array}{l}sqrt{5cdot 3}cdot sqrt{36cdot 5}cdot sqrt{2cdot 6}=sqrt{5cdot 3}cdot sqrt{3cdot 12cdot 5}cdot sqrt{2cdot 3cdot 2}=\=sqrt{5cdot 3}cdot sqrt{3cdot 2cdot 2cdot 3cdot 5}cdot sqrt{2cdot 3cdot 2}end{array})
На простые множители разложили. Что дальше? А дальше пользуемся свойством умножение корней и записываем все под одним знаком корня:
( displaystyle begin{array}{l}sqrt{5cdot 3cdot 3cdot 2cdot 2cdot 3cdot 5cdot 2cdot 3cdot 2}=sqrt{5cdot 5cdot 3cdot 3cdot 3cdot 3cdot 2cdot 2cdot 2cdot 2}=\=sqrt{25}cdot sqrt{81}cdot sqrt{16}=5cdot 9cdot 4=180end{array})
Вот и все, не так все и страшно, правда?
( displaystyle sqrt{15}cdot sqrt{54}cdot sqrt{10}=?)
Получилось ( displaystyle 90)? Молодец, все верно!
А теперь попробуй вот такой пример решить:
( displaystyle sqrt{4225}=?)
А пример-то – крепкий орешек, так сходу и не разберешься, как к нему подступиться. Но нам он, конечно, по зубам.
В уроке «Степень числа»
мы проходили, что возвести в квадрат число означает умножить число на само себя.
Кратко запись числа в квадрате выглядит следующим образом:
3 · 3 = 32 = 9
Но как быть, если нам нужно получить обратный результат?
Например, узнать, какое число при возведении в квадрат дало бы число «9»?
Запомните!
Нахождение исходного числа, которое в квадрате дало бы требуемое, называется
извлечением квадратного корня.
Извлечение квадратного корня — это действие, обратное возведению в квадрат.
У квадратного корня есть специальный знак.
Исходя из вычислений выше, нетрудно догадаться, что число, которое в квадрате дает «9»,
это число «3». Запись извлечения квадратного корня из числа «9» выглядит так:
√9 = 3
Читаем запись: «Арифметический квадратный корень из девяти». Можно опустить слово «арифметический».
Словосочетания «арифметический квадратный корень» и «квадратный корень» полностью равнозначны.
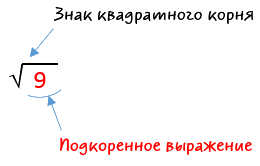
Число под знаком корня называют подкоренным выражением.
Подкоренное выражение может быть представлено не только одним числом.
Всё, что находится под знаком корня, называют подкоренным выражением. Оно может сожержать как числа, так и буквы.
Запомните!
Извлекать квадратный корень можно только из положительного числа.
-
√−9
= … нельзя извлекать квадратный корень из отрицательного числа; -
√64 = 8
-
√−1,44
= … нельзя извлекать квадратный корень из отрицательного числа; -
√256 = 16
Квадратный корень из нуля
Запомните!
Квадратный корень из нуля равен нулю.
√0 = 0
Квадратный корень из единицы
Запомните!
Квадратный корень из единицы равен единице.
√1 = 1
Как найти квадратный корень из числа
Квадратные корни из целых чисел, чьи квадраты известны, вычислить довольно просто.
Для этого достаточно выучить таблицу квадратов.
Чаще всего в задачах школьного курса математики требуется найти квадратный корень из квадратов чисел от
1 до 20.
Решение примеров с квадратными корнями
Разбор примера
Вычислить арифметический квадратный корень из числа.
- √81 = 9
- √64 = 8
- √100 = 10
Как найти квадратный корень из десятичной дроби
Важно!
При нахождении квадратного корня из десятичной дроби нужно выполнить следующие действия:
- забыть про запятую в исходной десятичной дроби и представить её в виде целого числа;
- вычислить для целого числа квадратный корень;
- полученное целое число заменить на десятичную дробь (поставить запятую исходя из
правила умножения десятичных дробей).
Более подробно разберем на примере ниже.
Разбор примера
Вычислить квадратный корень из десятичной дроби «0,16».
√0,16 =
По первому пункту правила забудем про запятую в десятичной дроби и представим ее в виде целого числа «16».
Нетрудно вспомнить, какое число в квадрате дает «16». Это число
«4».
√16 = 4
√0,16 = …
Вспомним правило умножения десятичных дробей.
Количество знаков после запятой в результате умножения десятичных дробей равняется сумме количества знаков после запятой каждой
дроби.
Т.е., например, при умножении «0,15» на
«0,3» в полученном произведении будет десятичная дробь с тремя знаками после запятой.
0,15 · 0,3 = 0,045
Значит, при вычислении квадратного корня
√0,16
нам нужно найти десятичную дробь, у которой был бы только один знак после запятой.
Мы исходим из того, что в результате умножения десятичной дроби на саму себя в результате должно было получиться
два знака после запятой, как у десятичной дроби «0,16».
Получается, что ответ — десятичная дробь «0,4».
√0,16 = 0,4
Убедимся, что квадрат десятичной дроби
«0,42» дает
«0,16».
Умножим в столбик «0,4» на
«0,4».
Рассмотрим другой пример вычисления квадратного корня из десятичной дроби. Вычислить:
√1,44 =
Представим вместо десятичной дроби «1,44» целое число
«144». Какое число в квадрате даст «144»?
Ответ — число «12».
122 = 144
√144 = 12
√1,44 = …
Так как в десятичной дроби «1,44» — два знака после запятой, значит в десятичной дроби,
которая дала в квадрате «1,44» должен быть один знак после запятой.
√1,44 = 1,2
Убедимся, что «1,22» дает в квадрате «1,44».
1,22 = 1,2 · 1,2 = 1,44
Квадратные корни из чисел
√2,
√3,
√5,
√6,
и т.п.
Не из всех чисел удается легко извлечь квадратный корень. Например, совершенно неочевидно, чему равен
√2
или
√3
и т.п.
В самом деле, какое число в квадрате даст «2»? Или число «3»?
Такое число не будет целым. Более того, оно представляет из себя
непериодическую десятичную дробь
и входит в
множество иррациональных чисел.
Что делать, когда в ответе остаются подобные квадратные корни? Как, например, в примере ниже:
√15 − 2 · 4 =
√15 − 8 =
√7
Нет такого целого числа, которое бы дало в квадрате число «7».
Поэтому, перед завершением задачи внимательно читайте её условие.
Если в задаче дополнительно ничего не сказано об обязательном вычислении всех квадратных корней, тогда ответ можно
оставить с корнем.
√15 − 2 · 4 =
√15 − 8 =
√7
Если в задании сказано, что необходимо вычислить все квадратные корни с помощью микрокалькулятора,
то после вычисления квадратного корня на калькуляторе
округлите результат до необходимого количества знаков.
Текст задания в таком случае может быть написан следующим образом:
«Вычислить. Квадратные корни найти с помощью калькулятора и округлить с точностью до
«0,001».
√15 − 2 · 4 =
√15 − 8 =
√7 ≈ 2,646